1+2+3+4+... = -1/12

This is a remarkable and hard to believe consequence in mathematics. If you were to sum the natural numbers all the way to infinity, you would get \(-\frac{1}{12}\).
$$ 1+2+3+4+5+6... = -\frac{1}{12} $$
This is just insane. It makes no sense that a series with an obviously increasing sum would end up being a small negative number.
If you were to take the \(nth\) partial sum of the sequence you would use this formula:
$$ \sum_{n}^{k=1} k=\frac{n(n+1)}{2}, $$
The \(nth\) partial sum increases without bound as \(n\) goes to infinity. This is because the sequence of partial sums fails to converge to a finite limit. The series does not have a limit[1].
One can however manipulate the the value to yield a number of interesting results. Some of these have applications in fields such as complex analysis, quantum field theory and string theory.
Proof
I recommend watching the Numberphile video which shows the proof for this. I won't do it justice.
Here is my attempt at explaining it. We want to solve for this:
$$ S = 1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + ... = ? $$
We are going to do this by relating it to another alternating series:
$$ 1 - 2 + 3 - 4 + 5 - 6 + 7 - 8 + ... = $$
This one is easier to work with and there are tricks for getting values for this divergent series.
We can do this by subtracting:
$$ 4S_2 = 4 + 8 + 12 + 16 ...$$
We also know that this alternating series:
$$ -3S_2 = 1 - 2 + 3 - 4 + 5 - 6 + 7 - 8 + ... $$
takes on the form:
$$ \frac{1}{(1+x)^2} $$
Where \(x=1\). So we can rewrite it to look like this:
$$ -3S_2 = 1 - 2 + 3 - 4 + 5 - 6 + 7 - 8 + ... = \frac{1}{(1+1)^2} = \frac{1}{4} $$
Now if we divide both sides by -3 we get \(-\frac{1}{12}\).
The fact that a clearly growing series can somehow end up as a negative number is just beyond intuition. The explanation for this lack of logic is that we don't really fully understand infinity. We know that if we stop the series at any point and measure it, it will likely be a very big number, but what happens at infinity? We just don't know.
Another way to think about it is that when you include all of the terms, all the way out to infinity, the sum truly equals \(-\frac{1}{12}\).
Let's graph it
I plotted this sum on Wolfram Alpha to see what it looks like:

As you can see the sum clearly goes towards infinity. So how are we getting this \(-\frac{1}{12}\)? What if we look at the negative values of \(n\) too? (Click here to see it on Wolfram Alpha
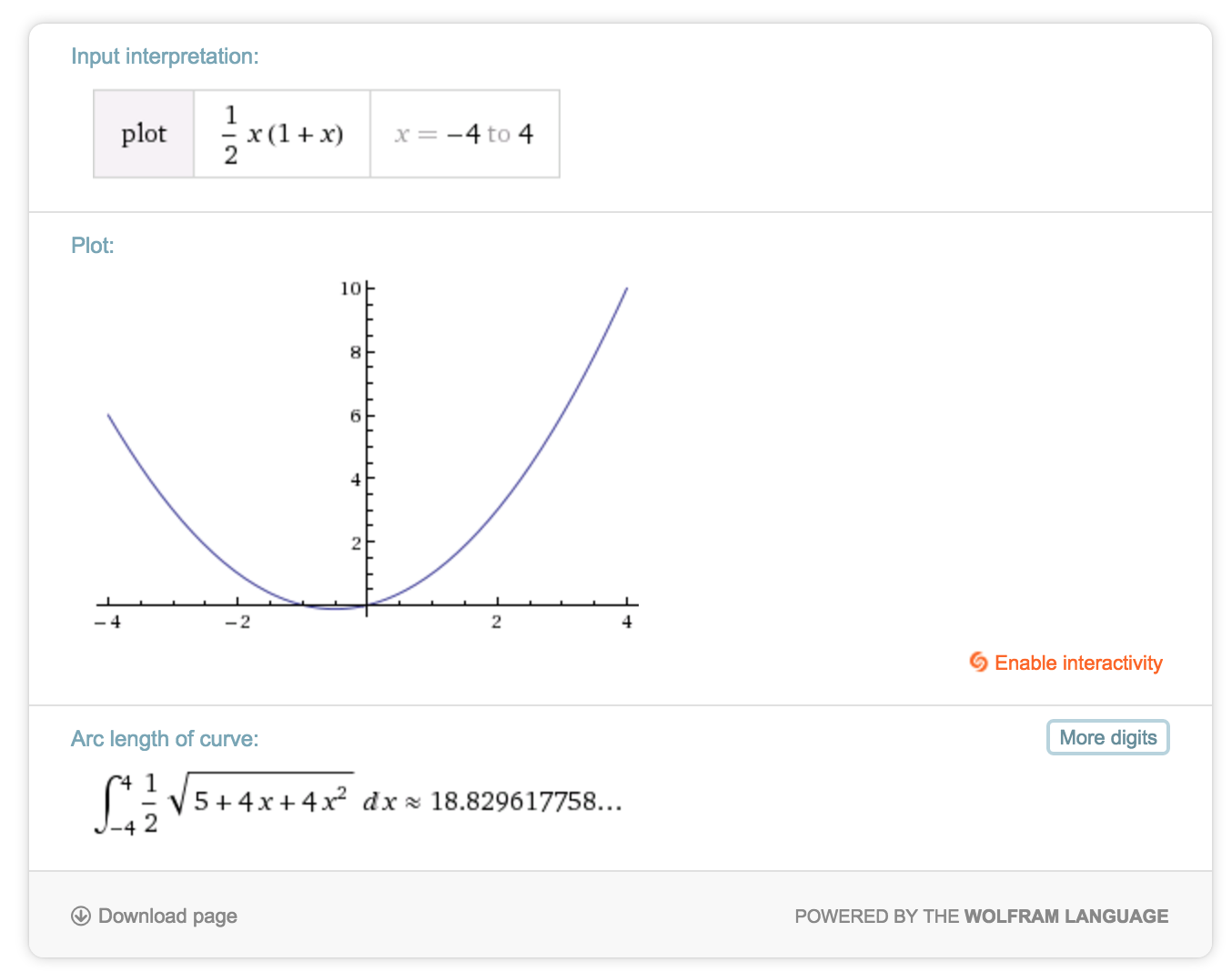
Do you see that portion of the graph that dips below the line? As both sides of the series continue to infinity that small portion of the graph below the line remains small. If you use calculus to determine its size:
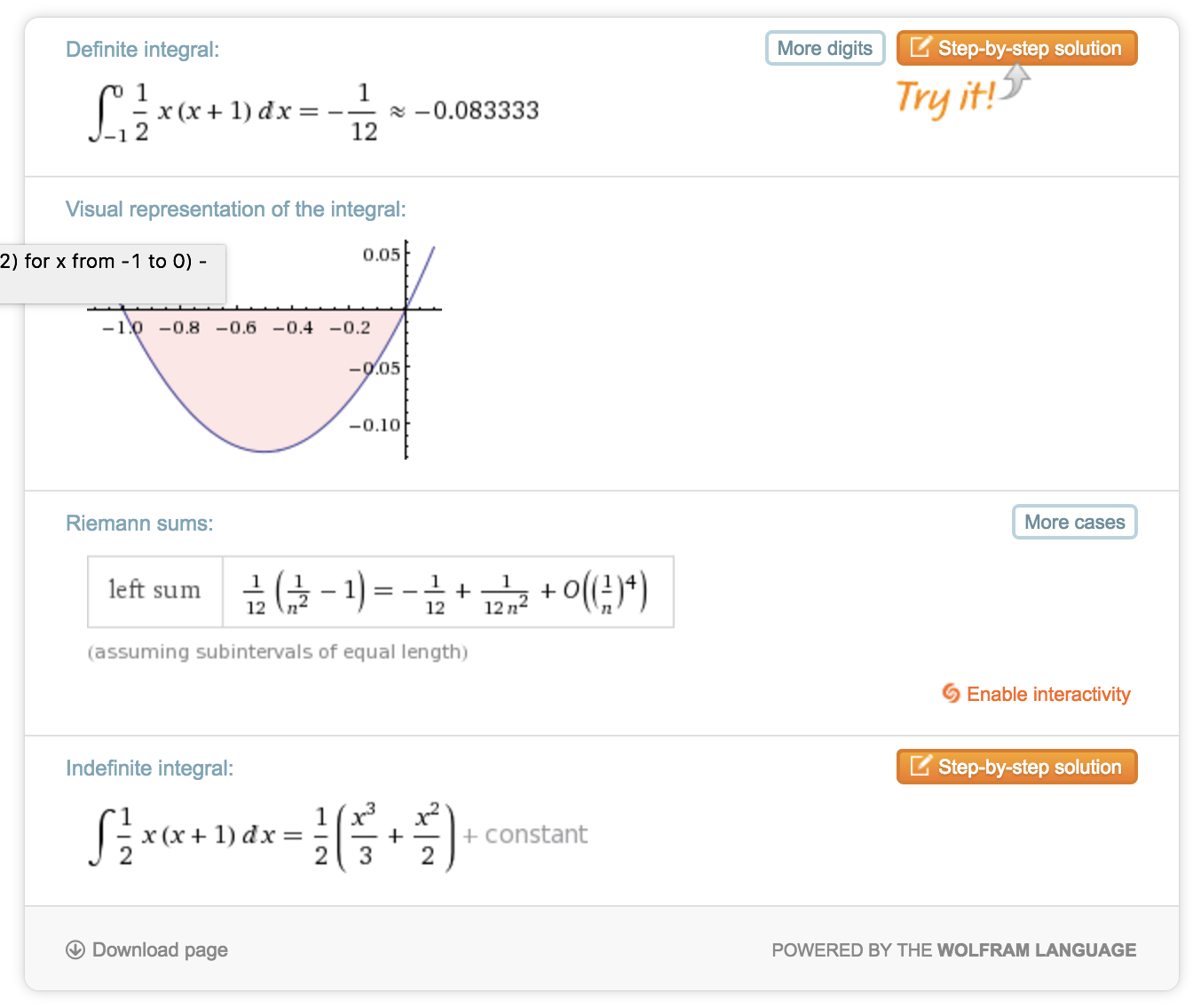
And there we go. The area under the curve is equal to \(-\frac{1}{12}\). That would help explain the result[2] but it still doesn't make any sense.
In Physics
In string theory with the study of bosons scientists look to compute the energy levels of a string. Strings vibrate and create harmonics which can be measured using this divergent series:
If the fundamental oscillation frequency is \(\omega\) then the energy in an oscillator contributing to the \(nth\) harmonic is \(\frac{nh\omega}{1}\). So using the divergent series, the sum over all harmonics is:
$$-\frac{h\omega(D-2)}{24}$$
It is also seen in computing the Casimir force for a scalar field in one dimension. I'm not familiar with how exactly this works, but the result is a constant of \(-\frac{1}{12}\).
This is just one of many divergent series that are useful in science. It is also a great example of a non-intuitive result in math. I still can't fathom how this trickery works. An infinitely increasing sum should be infinitely increasing right? So how can we assign a value to it?
